There are times and places in the universe that experiments cannot reach, and may never be able to reach. What exactly goes on inside black holes, and what happened in the first fractions of a second after the Big Bang, are matters of pure theoretical speculation.
For two decades, Silke Weinfurtner, a physicist at the University of Nottingham in the United Kingdom, has been designing “analogue gravity” experiments using fluids that mimic systems like black holes and the early universe. For example, she has detected sound waves in fluids that resemble Hawking radiation — the subtle heat emitted by black holes that holds deep implications for what happens to information in the universe. Another fluid effect she studies mimics how the seeds of cosmic structure were sown in the first moments after the Big Bang.
Weinfurtner and other analogue gravity experimenters make the controversial claim that by studying fluids here on Earth, we can glimpse truths about the physics of the most extreme and far-off phenomena in the universe. Certainly, the approach has critics, who say that the similar math governing these systems, although surprising, isn’t enough to allow one to stand in for the other. For Weinfurtner, the startling mathematical similarities between them that emerge under certain conditions can be exploited. “It’s mind-blowing how physics really works,” she told me recently. “It just seems like nature threw us a bone when things are really hard.”
The hard physics that’s out of experimental reach involves the unknown, quantum nature of gravity. In Albert Einstein’s general relativity, gravity comes about when matter bends the fabric of space-time. But physicists seek the more fundamental, quantum theory of gravity that underlies Einstein’s picture; it’s this quantum gravity theory that governs extremes like the Big Bang and black holes. And one way to inch toward this complete theory is to study quantum fluctuations in the space-time fabric. Quantum field theory describes particles as excitations in underlying fields, like ripples in a pond. These fluctuations are then layered on top of a curved space-time background. “A lot of new cool physics emerges,” said Weinfurtner.
And, mysteriously, analogous cool physics arises in more familiar physical systems.

Weinfurtner meets with graduate students in her office.
Philipp Ammon for Quanta Magazine
Introduction
The Canadian physicist Bill Unruh kicked off analogue gravity research in the 1970s by drawing a comparison between the sound of a screaming fish descending a waterfall and light that’s trapped in the warped space-time around a black hole. This memorable mathematical equivalence eventually led Unruh and others to build makeshift analogue experiments in an undergraduate engineering lab. It was there, in the University of British Columbia’s “rusty hut” in the late 2000s, that Weinfurtner, a postdoctoral researcher originally from Germany, began trying to tame these fluid systems.
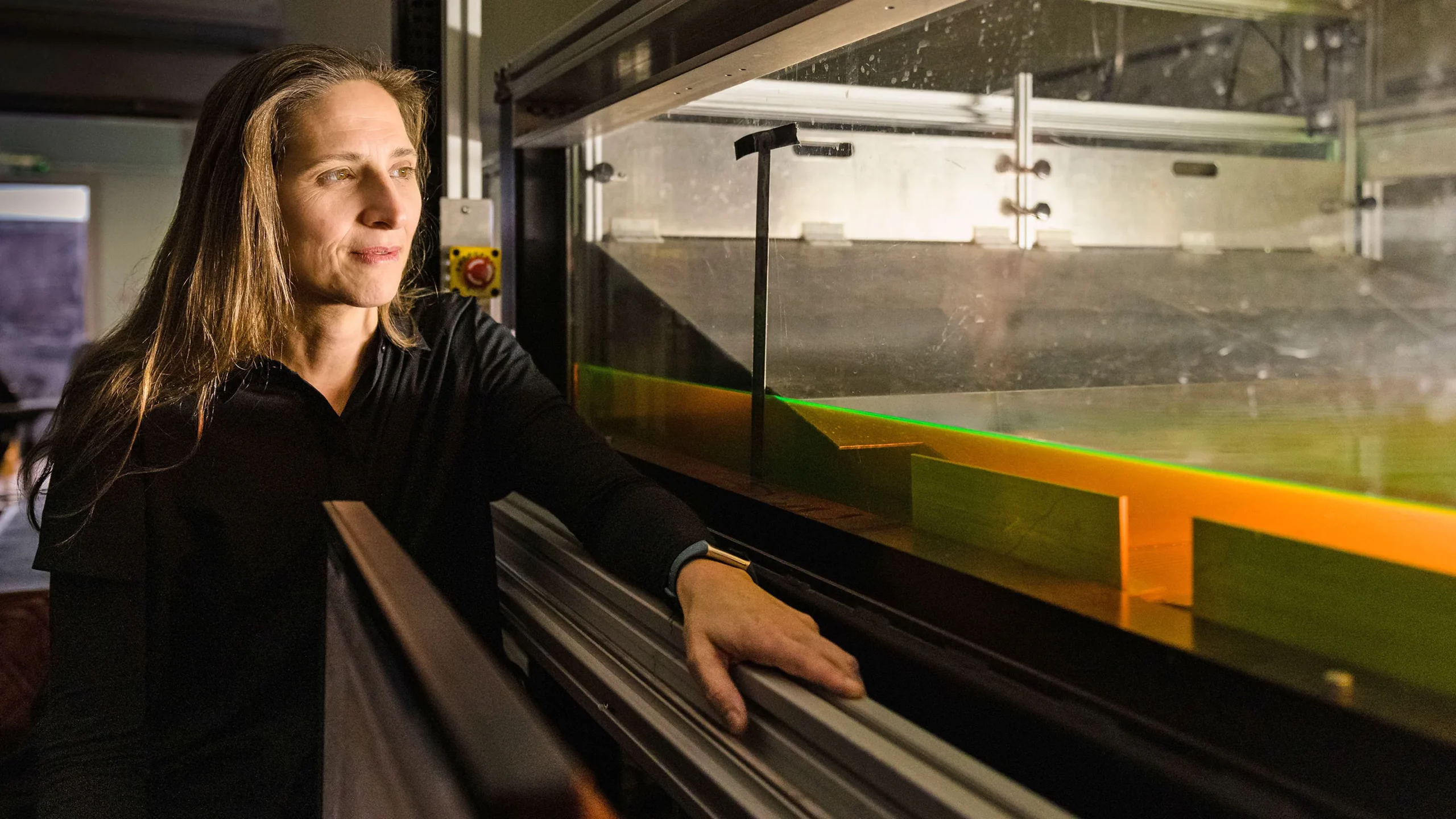
Ever since, Weinfurtner has been building increasingly sophisticated analogue experiments, and today she is the leader of the UK’s foremost analogue gravity research group. When I visited one of her laboratories recently, green laser light reflected off waves on the surface of what amounted to an oversize bathtub. A vortex swirled down the drain in the center of the tub, dragging the regularly spaced ripples around it in a snail-shell-like pattern. The ripples drifted past an artificially created point of no return — the analogue of a black hole’s inescapable event horizon. “It is a giant bathtub, but it’s a very special one,” Weinfurtner told me.
In another, new lab, superfluid helium simmered inside a silvered bell jar. There, mesmerizing effects analogous to quantum gravitational phenomena in the early universe “unfold in front of your eyes,” she said. “You get a feeling of how weird it is.”
For four hours we wandered around these labs and strolled the lakeside campus in the autumn sunshine, squawking geese and all, as we discussed Weinfurtner’s research and its interpretations. “What’s up there is not so strange,” she said, referring to the inaccessible realms of the cosmos. “I’m looking for a deeper truth beyond one system.”
The interview has been condensed and edited for clarity.
How do you make a tub of fluid behave like the early universe?
Let’s say this square coffee table is our superfluid helium tank. I can create a surface wave that propagates on the table surface. If I make the wave speed smaller and smaller, driving it toward zero, the wave will never reach the boundary. So for this wave, this table looks infinitely big. This is like cosmic inflation, the very rapid expansion of space that theorists think happened shortly after the universe’s birth. During inflation, neighboring particles will appear to be moving away from each other faster than the speed of light, and so the universe seems infinitely big.

The fluid analogue of a black hole forms as water swirls down a drain at the bottom of a tub. Green lasers reflect off the water’s surface.
Philipp Ammon for Quanta Magazine
Introduction
Recently, you simulated how energy spread from the quantum field that drove cosmic inflation to particles of matter in the early universe. How does this work?
During inflation, you get fluctuations in the quantum fields that fill space-time, and these are stretched along with the expansion of the universe. They become imprinted on the space-time background and become seeds for the large-scale structure of our universe. Then, after inflation, these “frozen modes” need to unfreeze so that particles can start interacting with each other again. For this, cosmologists use a mechanism called preheating, which takes these fields that were rapidly expanding but were never really talking to each other and creates an environment where the fields start interacting with each other.
You can re-create this in fluids. You tap the surface of a fluid, or shake it, to make a wave with a certain frequency. If the wave isn’t too tall, then this wave doesn’t interact with other waves of different frequencies — you can try this in the bathtub. But if the amplitude gets high, then the different frequencies interact.
In the mathematics, there’s the part of the wave equation where every wave is just minding its own business. And then there’s a very, very large number of extra terms that tell us how each frequency interacts with another frequency. In principle we have almost infinitely many terms which we could consider, so we have to pick some terms to focus on; otherwise you can’t solve the equation. We make assumptions such as that you don’t have dissipation in your system, and that the fields are weakly interacting, and so forth.
In our experiments, we test whether these approximations are reasonable. Our system has all the interaction terms built in. And indeed, we find that all of the approximations cosmologists use carry over. So these assumptions are robust; they withstand physical scrutiny in our system.
It’s still surprising that you can tell anything about what’s going on in the early universe, or black holes, by studying fluids moving in a big bathtub. What’s underpinning all of this?
The mathematical equations describing the physics in the bathtub also describe the physics around the black hole, or in the early universe. One cannot say these are the same systems. The fluid system is a rather complex physical system, and also the mathematics is quite complicated; it’s described by the so-called Navier-Stokes equations for fluids. But with certain assumptions that can be replicated in experiments, you can reduce this complexity significantly, and you’re left with an equation that can be mapped to how quantum or classical fields propagate around black holes or in the early universe; I find the same equation, to a good approximation. So it’s all resting on a mathematical analogy.
I’m saying, well, if the equations are the same, the physics should be the same. I want to understand this bit of mathematics; I don’t care if it was discovered first for black holes or other systems. There’s a deeper truth beyond a particular system. And this is what we’re after.
So this rests on the idea of universality, that there is common behavior over and above the microscopic details of the system.
I’m not interested in probing the microphysics of these fluid systems, I’m interested in the macroscopic emergent behavior. Why are the macroscopic behaviors of two very different systems — field theories on curved space-time and excitations in fluids and superfluids — the same? I don’t know. Physics has this remarkable feature that it repeats itself.
So maybe we shouldn’t be giving up. We can gain experience with these more accessible systems and build up the expertise to go beyond that.
Giving up on what?
Observations of quantum fields in curved space-time. Hawking radiation from black holes, for example, has remained a theoretical field of research, where people have more and more confidence in calculating things — there have been developments in putting the whole field on a more rigorous mathematical formulation. But it has remained undetectable. So it’s been put in a drawer labeled “Theory. Probably correct. Let’s move on.”

A superfluid helium tank that’s used to simulate events in the early universe sits on a table that nullifies exterior vibrations and waves.
Philipp Ammon for Quanta Magazine
Introduction
But you’re trying to open up that drawer?
It still seems an open problem to me. And because of this lack of experimental feedback, we don’t understand quantum field theory in curved space-time well enough. The textbooks we have are pure theory. Only through experiments can we get to a deeper level.
When we solve equations of quantum fields in curved space-time, there are often leaps of faith when we make approximations. There’s ambiguity built in. For example, there is a big question mark around which initial state to choose when you prepare your quantum field. And analogue experiments can fill this gap. We can put many of those approximations to the test.
Is it hoped that this will lead toward direct experiments, or can analogues replace them?
We have no clue how we could actually measure a black hole’s Hawking radiation. The analogue experiments are looking at effects like these and developing a protocol for how to extract them, measure them, verify them. We’re embedding these kinds of effects in a physical reality, adding all the extra layers needed that could lead up to a detection.
I’m asking: How can these analogues feed back onto the theoretical side? Could this inject a new way of thinking about experimental realities, so that in five, 10 or maybe 50 years, we could actually see some of these effects [like Hawking radiation] directly in experiments? Let’s take everything onboard that we learned from these wonderful theoretical outcomes, and let’s load that onto these high-tech direct experiments. So analogues are a vital steppingstone in the right direction of putting experiments in the heart of quantum fields and gravity.
Is it possible to use analogue experiments to do more than test the approximations used in these theories?
You could also look for new physical effects that you missed because you did all these approximations. Here we come with this messy analogue system, and then you can ask: Well, I see these additional effects in my analogue system; does it make sense that this would also exist beyond the analogue?
The dream would be that you use the experiments to “calculate” something — to simulate effects in our analogues beyond what you calculate analytically. But how much can you trust that the outcome of this simulator also holds in the target system that it is analogous to? I don’t have an answer, but it’s a question that keeps me up at night.

Silke Weinfurtner poses in front of the George Green Library on the University of Nottingham campus.
Philipp Ammon for Quanta Magazine
Introduction
Critics of analogue experiments might balk at the idea of discovering something new about gravity by looking at a fluid in a lab.
I would waste my time trying to persuade everyone to like analogue gravity. All I can do is produce the best possible outputs, make sure that there is a young, vibrant community, and be honest — without overclaiming. Some people will never change their mind about analogue experiments, but I can live with that.
It seems like this is a very different way of approaching physics, or even a new kind of knowledge acquisition — beyond, say, direct experiments, pen-and-paper theory, and computer simulations.
In physics we usually take a particular system and look for all the physical processes in that system. In analogue gravity, it’s different — you’re interested in seeing Hawking radiation emerge from some other system. The medium is flexible. It’s a bit like art. If you’re an artist, you can choose to paint, be a sculptor, or make a video installation. You choose the medium, whatever it takes you to realize the idea you have in your mind. And this freedom is what creates unease in some of my colleagues.
I believe that experimental physics is about bringing abstract ideas to life, like artists. But my system is not fixed. And this also makes it accessible, because the systems we’re using sometimes are commonplace. That allows everyone to dive in and to live it. We have just built an art lab next door to our black hole lab to create space for outreach, allowing artists and curious people to work with us. The goal is to create an inclusive research community with an open door to people who are usually on the outside. The idea is to create fun and excitement about our research, and for us to learn from artists and school kids.
The physics around black holes is something very abstract, very unapproachable. And then a visitor says: “Wait a second, that’s a bathtub. So I should be able to understand.” And that allows everyone to start asking questions.
